Accurate dosage calculation is a key skill required to safely dispense drugs. Ensuring each patient is given the right medicine and the right dose is everyone’s responsibility which makes this section relevant to everyone in nursing care – even if you are not the individual who initially calculated the dosage.
We all play a vital role in checking the dose given to the patient and any of us has the potential to catch an accidental error that might otherwise lead to the wrong dose being provided to those in our care. In this section, we’ll look at how you can calculate the amount of medicine to give a patient using different techniques.
Mental arithmetic for drug calculation
There are different ways you might use mental arithmetic to calculate dosage. Let us consider an example.
A child has been prescribed 5 mg of a drug that is available in liquid form as 2 mg per ml.
In this case, if we have 2 mg per ml, it is easy to see that 1 mg would be found in half the volume. So, 1 mg is found in 0.5 ml of solution.
So, if there is 1 mg of active drug in 0.5 ml, we can multiply 0.5 ml of the solution by five to get our answer (as we want 5 mg of the drug). 0.5 multiplied by five is equal to 2 and a half milliliters.
Universal formula for drug calculation
The universal formula for drug calculation is also known as Desired Over Have Formula.
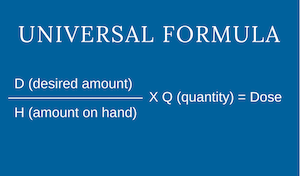The desired amount (D) is the dose prescribed
The amount on hand (H) or the amount you “have” is the available dose or concentration.
The quantity (Q) in the form and amount in which the drug is supplied.
To calculate the dose, take the desired amount and divide it by the amount on hand, then multiply it by the quantity. Below are the drug calculation examples in different forms
Oral dose calculation
Cephalexin (Keflex) 750 mg P.O. every 12 hours is ordered. The pharmacy stocks 250 mg tablets. How many tablets should be administered per dose?
Intravenous (IV) dose calculation
An order for digoxin 0.5 mg IV daily is placed. Digoxin 0.25 mg/mL is available from the pharmacy. How many mL will you need to administer a 0.5 mg dose?
Subcutaneous dose calculation
Heparin 7500 units subcutaneous every 12 hours is ordered. The pharmacy provides a heparin vial with a concentration of 5000 units/mL. How many mL will you need to administer 7500 units?
Dimensional Analysis Method for drug calculations
An order placed by a provider for lorazepam 4 mg IV PUSH for CIWA score of 25 or higher, follow CAGE Protocol for subsequent dosages based on CIWA scoring.
- The clinician has 2 mg/mL vials in the automated dispensing unit.
- How many milliliters are needed to arrive at an ordered dose?
- The desired dose os placed over 1 remember, (x mL) = 4 mg/1 x 1 mL/2 mg x (4)(1)/2 x 4/2 x 2/1 = 2 mL, keep multiplying/dividing until the desired amount is reached, 2 mL in this example.
- Notice, the fraction was set up with milligrams and milligrams strategically placed, so units could cancel each other out, making the equation easier to solve for the unit desired or milliliters. The answer makes sense, so work is done.
Zeros can be canceled out in the same way as units. For example:
- 1000/500 x 10/5 = 2, the 2 zeros in 1000 and 2 zeros in 500 can be crossed out since like units in numerator and denominator, leaving 10/5, a much easier fraction to solve, and the answer makes sense.
We have addressed zeros, and now let us look at 1.
- If one multiplies a number by a 1, then the number is unchanged.
- In contrast, if you multiply a number by zero, the number becomes zero.
- Examples listed below are as follows: 18 x 0 = 0 or 20 x 1 = 20.
Ratio and Proportion technique
The Ratio and Proportion Method has been used in drug estimates for many years and is one of the oldest. This relationship has no influence on addition principles, which is a problem-solving strategy. Only multiplication and division are used to solve a ratio and percentage problem. Using a fraction or colon format, the following example will provide a better explanation:
A provider orders lorazepam 4 mg IV Push now for a CIWA score of 25. There are 2 mg/mL vials on hand. How many milliliters are required to carry out the ordered dose?
- Have on hand / Quantity you have = Desired Amount / x
- 2 mg/1 mL = 4 mg/x
- 2x/2 = 4/2
- x = 2 mL
One would use H: V: :D: X and multiply means DV and Extremes HX in colon format.
- Hx = DV, x = DV/H, 2:1::4:x, 2x = (4)(1), x = 4/2, x = 2 mL
How to check you your drug calculations?
Remember to double-check your work once you’ve finished your calculations. Here are some examples of how you could do this:
- Repeat the calculation
- Ask a colleague to check your answer
- Try to calculate the answer again using a different method
- Check against the recommended dose range (e.g., using the British National Formulary)
- Look for unusually big or small answers.
Nursing revalidation provides an interactive drug calculation course. This course is suitable for any healthcare practitioner who may be involved in the administration of medicines to patients. This drug calculation course will cover formulas to convert between units and calculate doses, as well as covering basic math skills that will be needed. Join the course and enhance your drug calculation skills.
